Unit 9: Torque & Rotational Mechanics
Practice Problems

Note: problem difficulty is ranked using a star system.
(*) One-star problems are fundamental to the unit, and can be done relatively quickly. Use these problems to introduce yourself to the material.
(**) Two-star problems are more difficult, and require an understanding of one or two key concepts. Use these problems to test your understanding of the material.
(***) Three-star problems are the most difficult, and require some creative thinking in addition to a deep familiarity with multiple key concepts. Use these problems to challenge yourself; if you can complete one of these, you’re on your way to mastering the material.
*Q9.1) Consider a pencil fixed in-place at the eraser. How much torque is exerted by the force (in blue) in each of the cases shown below? Consider counterclockwise torques to be positive.
a)
b)
c)
d)
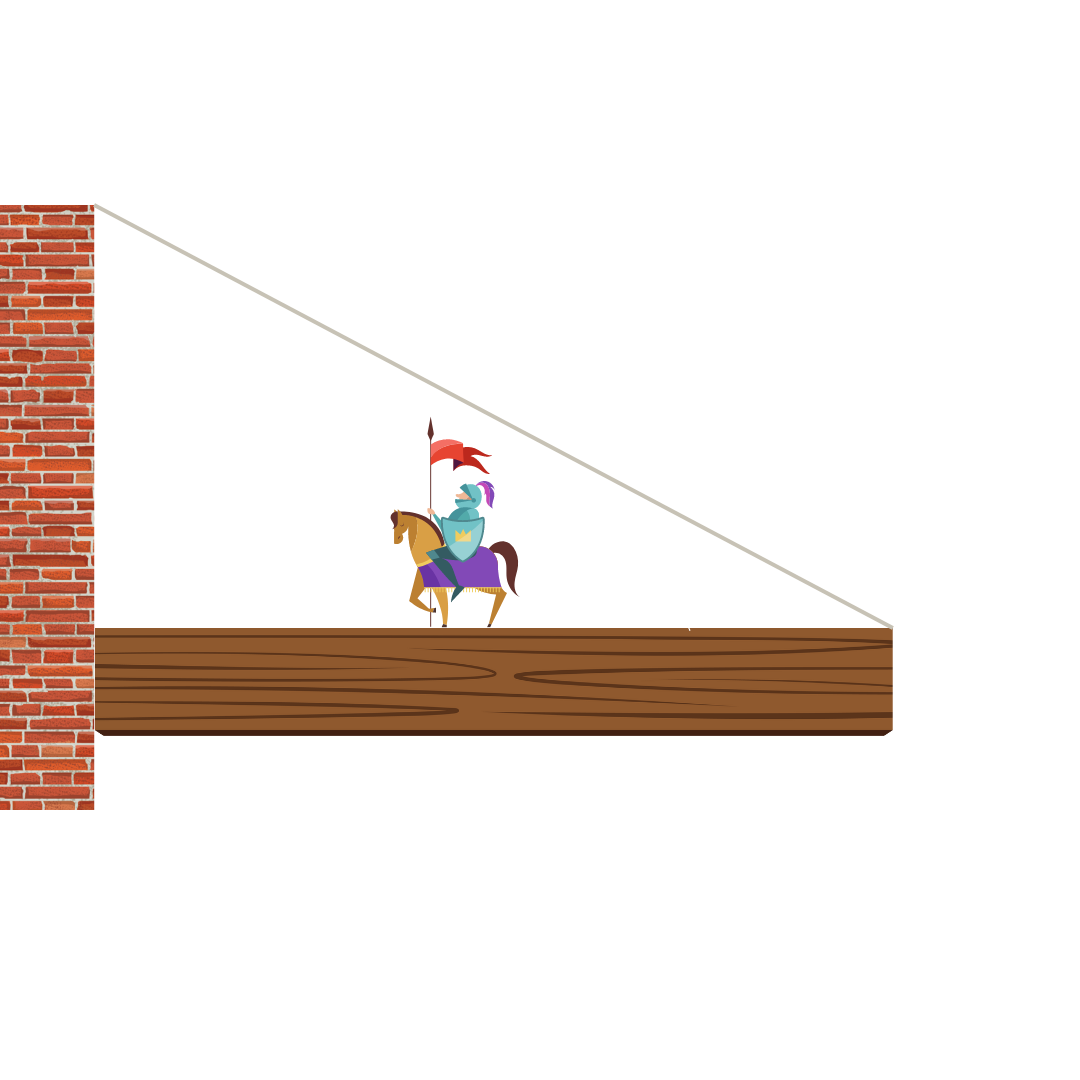
**Q9.2) A knight and a horse stand at the midpoint of a stationary drawbridge. The horse has a mass of 600kg, the knight has a mass of 200kg, and the bridge has a mass of 100kg. Calculate the tension in the supporting cable shown below.
**Q9.3) A 20kg mass rests on a 4kg plank supported by a wooden block and a bathroom scale (as shown below). If the plank is 2m long and the bathroom scale reads 80N, how far from the left end of the plank is the mass located?
**Q9.4) You wrap some rope around a cylinder of mass 20kg and radius 10cm, then pull on the rope with a force of 200N. If you maintain this force for 10 seconds and the cylinder starts from rest, what length of rope unwinds from the cylinder? Note: the moment of inertia of a solid cylinder is given by I = ½mr².
***Q9.5) A ladder of length 3m and mass 10kg leans against a frictionless wall at a 60 degree angle from the horizontal (as shown below). A man stands on the ladder so that his center-of-mass is positioned 1m from the base of the ladder (measured along the ladder). If the coefficient of static friction between the ladder and the floor is 0.3, what maximum mass can the man have without causing the ladder to slip?
***Q9.7) Consider the uniform door shown below. It has a mass of 60kg and is connected to the wall via two hinges on its left side that are separated by 1.4m. The force exerted on the door by the upper hinge acts perfectly horizontally. Calculate the magnitude and direction of the forces exerted by each hinge on the door.
***Q9.6) What’s the maximum height above the tabletop you could push on the glass of water shown below without causing it to tip? Assume the glass of water is perfectly cylindrical and uniform in density, and that the coefficient of static friction between the glass and the table’s surface is 0.3. You may also assume your force is exerted parallel to the tabletop.